 Crystallographic Topology 101
Crystallographic Topology 101

Color Groups
The color crystallographic groups (Schwartzenberger, 1984), which have both symmetry and antisymmetry operators, provide a convenient formalism for describing group/normal-subgroup relationships. Each group of a crystallographic bicolor group family describes one index-2 group/subgroup pair of regular crystallographic groups while the tricolor members describe index-3 pairs etc.
There are 1651 groups in the complete bicolor space group family, 230 of which are the regular space groups with black symmetry operators, another 230 have simultaneously black and white (gray) symmetry operators, and the remaining 1191 nontrivial bicolor space groups have mixed black and white operators. Of the 1191, 674 groups contain no anti-translations while 517 have anti-translations (i.e., have bicolor Bravais lattices). When all translations are removed, the bicolor space groups produce 122 bicolor point groups, 32 regular point groups, 32 gray point groups, and 58 with mixed black and white symmetry operators. Our immediate interest is in the 58 membered set of groups and their occurrence in the 1191 nontrivial bicolor space groups.
The bicolor groups are also called Shubnikov, Heesch, or magnetic groups in the crystallographic literature. There is an additional set of 7 tricolor elliptic 2-orbifolds needed to define the index-3 group/normal-subgroup relations of Fig. A.2. These may be derived from the tricolor groups given in a "Table of Colored Groups as Extensions of the Crystallographic Groups" in Shubnikov and Koptsik (1974). Index-4 subgroups might also be included but are not needed in the present application.
Antisymmetry
The concept of antisymmetry was introduced by Heesch (1929) and Shubnikov (1945). Black and red drawings of the 58 bicolor point groups and 1191 bicolor 3-Euclidean groups and tables of all the Wyckoff positions are given in Koptsik (1966). In the Koptsik drawings, the black symmetry elements denote conventional symmetry operators and the red elements antisymmetry operators. Bicolor groups are called magnetic groups when used to describe simultaneously the arrangement of atoms (regular symmetry) and the up/down magnetic spin vector orientations (antisymmetry) for magnetic atoms in a crystal (Opechowski and Guccione, 1965).
Each element of a bicolor crystallographic group has an associated even or odd binary parity flag, determined by the product of group generator parities that produce the element. Since the group generators for space groups are given explicitly in the crystallographic space group nomenclature, the bicolor space group nomenclature is given by simply placing flags, such as *, on the antisymmetry operators in the group name (see Table A.1).
There are an equal number of even parity (black) and odd parity (white) group elements in a bicolor group L with a maximal normal subgroup, H, of L defining the set with even parity. The remaining members of the group L have a negative parity and a color graphical representation. A "color blind" reading of the complete set of color and black operators in L produces a black supergroup, G, of H where G and H are both regular non-color groups. Tricolor groups are related to the bicolor groups by use of modulo 3 rather than modulo 2 parity. For compatibility with the bicolor cases, we define two of the three colors to be a single color and the modulo 3 = zero parity color, black.
The antisymmetry operators within a color orbifold describe the sheeting characteristics of an orbifold cover since a suborbifold is just a partial unfolding of a parent orbifold. The orbifolding is done using only the regular (black) symmetry operators and cannot occur along antisymmetry (color) elements.
Bicolor Point Groups
A list of the crystallographic point groups and their derivative bicolor point groups is given in Table A.1. The antisymmetry generators are denoted by a asterisk (*) in the point group symbol.
| Type | Orbifold | Point Group | Bicolor Point Groups | ||||
|---|---|---|---|---|---|---|---|
| a | 1 | 1 | |||||
| b | 0 | 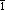 | 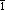 * * | ||||
| c | 1' | m | m* | ||||
| d | 22 | 2 | 2* | ||||
| f | 21' | 2/m | 2*/m. | 2./m* | 2*/m* | ||
| g | 222 | 222 | 2*2*2. | ||||
| h | 2'2' | mm2 | m*m.2* | m*m*2. | |||
| j | 2'2'2' | mmm | m*m.m. | m*m*m. | m*m*m* | ||
| d | 44 | 4 | 4* | ||||
| e | 20 |  |  * * | ||||
| f | 41' | 4/m | 4*/m. | 4./m* | 4*/m* | ||
| g | 422 | 422 | 4*2.2* | 4.2*2* | |||
| h | 4'4' | 4mm | 4*m*m | 4.m*m* | |||
| i | 22' |  2m 2m |  *2*m. *2*m. |  *2.m* *2.m* |  .2*m* .2*m* | ||
| j | 4'2'2' | 4/mmm | 4./m*m.m. | 4*/m.m*m. | 4*/m*m*m. | 4./m.m*m* | 4./m*m*m* |
| d | 33 | 3 | |||||
| f | 31' | 3/m= |  * * | ||||
| g | 322 | 32 | 32* | ||||
| h | 3'3' | 3m | 3m* | ||||
| j | 3'2'2' |  m2 m2 |  *m*2. *m*2. |  *m.2* *m.2* |  .m*2* .m*2* | ||
| d | 66 | 6 | 6* | ||||
| e | 30 |  |  * * | ||||
| f | 61' | 6/m | 6*/m. | 6./m* | 6*/m* | ||
| g | 622 | 622 | 6*2*2. | 6.2*2* | |||
| h | 6'6' | 6mm | 6*m*m. | 6.m*m* | |||
| i | 23' |  m m |  *m. *m. |  .m* .m* |  *m* *m* | ||
| j | 6'2'2' | 6/mmm | 6./m*m.m. | 6*/m.m*m. | 6*/m*m*m. | 6./m.m*m* | 6./m*m*m* |
| k | 332 | 23 | |||||
| l | 32' | m | m*3 | ||||
| m | 3'3'2' |  3m 3m |  *3m* *3m* | ||||
| n | 432 | 432 | 4*32* | ||||
| o | 4'3'2' | m m m | m*3m. | m.3m* | m*3m* | ||
Color Elliptic 2-Orbifold Table
Column 3 of Table A.2 lists the 58 bicolor [and 7 tricolor (# in column 1)] crystallographic point group/normal subgroup pairs, G:H, for the 32 crystallographic point groups, with the corresponding bicolor (and tricolor) point group, L, listed in Column 4. The negative parity generators are denoted by a * for mod 2 antisymmetry or a # for mod 3 antisymmetry as a suffix in Column 4. All group notation is in international crystallographic form.
The notation 9A jf12 in Col. 1 of Table A.2, for example, denotes bicolor
topology family 9A, orbifold family "j", and suborbifold
family "f". The number 12 at the end of the identifier is the order of
the group,  m2, from which the orbifold is derived.
m2, from which the orbifold is derived.
The orbifold for line 9A jf12 has singular set 3'2'2' consisting of one
3-fold axis and two 2-fold axes all lying in intersecting pairs of
mirrors. The suborbifold
31' has one 3-fold axis (3) which intersects a mirror plane (1'). The
crystallographic point group and positive parity subgroup are
 m2
and
m2
and  , respectively.
, respectively.
The bicolor group in line 9A jf12 is generated by the starred negative parity
generators, m and 2,
plus the positive parity generator,
 (= 3/m),
with the position of the generator symbol within the group name,
(= 3/m),
with the position of the generator symbol within the group name,
 *m*2, denoting its geometric orientation relative to the
hexagonal crystallographic family coordinate system axes.
*m*2, denoting its geometric orientation relative to the
hexagonal crystallographic family coordinate system axes.
We note in passing that  is identical
with 3/m. The unfortunate
original choice of
is identical
with 3/m. The unfortunate
original choice of  instead of 3/m
for crystallographic nomenclature
by the founding fathers leads to considerable non-uniformity in Table A.2 and
elsewhere.
instead of 3/m
for crystallographic nomenclature
by the founding fathers leads to considerable non-uniformity in Table A.2 and
elsewhere.
| Identifier (a) (b) |
Orbifold:Suborbifold Singular Set Notation |
Point Group:Subgroup (generators) |
Color Group (generators) |
|
|---|---|---|---|---|
| 1 | ba02 | 0:1 | 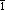 :1 :1 | 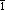 * * |
| 2 | ca02 | 1':1 | m:1 | m* |
| 3A | da02 | 22:1 | 2:1 | 2* |
| #3A | da03 | 33:1 | 3:1 | 3# |
| 3B | dd04 | 44:22 | 4:2 | 4* |
| 3B | dd06 | 66:33 | 6:3 | 6* |
| #3B | dd06 | 66:22 | 6:2 | 6# |
| #4A | eb06 | 30:0 |  : :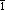 |  # # |
| 4B | ed04 | 20:22 |  :2 :2 |  * * |
| 4C | ed06 | 30:33 |  :3 :3 |  * * |
| 5A | fb04 | 21':0 | 2/m: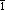 | 2*/m* |
| 5B | fc04 | 21':1' | 2/m:m | 2*/m. |
| #5B | fc06 | 31':1' |  :m :m |  # # |
| 5C | fd04 | 21':22 | 2/m:2 | 2./m* |
| 5C | fd06 | 31':33 |  :3 :3 |  * * |
| 5C | fd08 | 41':44 | 4/m:4 | 4./m* |
| 5C | fd12 | 61':66 | 6/m:6 | 6./m* |
| 5D | fe08 | 41':20 | 4/m: | 4*/m* |
| 5D | fe12 | 61':30 | 6/m: | 6*/m* |
| 5E | ff08 | 41':21' | 4/m:2/m | 4*/m. |
| 5E | ff12 | 61':31' | 6/m: | 6*/m. |
| #5E | ff12 | 61':21' | 6/m:2/m | 6#/m |
| 6A | gd04 | 222:22 | 222:2 | 2.2*2* |
| 6A | gd06 | 322:33 | 32:3 | 3.2* |
| 6A | gd08 | 422:44 | 422:4 | 4.2*2* |
| 6A | gd12 | 622:66 | 622:6 | 6.2*2* |
| 6B | gg08 | 422:222 | 422:222 | 4*2.2* |
| 6B | gg12 | 622:322 | 622:32 | 6*2.2* |
| 7A | hc04 | 2'2':1' | 2mm:m | 2*m.m* |
| 7B | hd04 | 2'2':22 | 2mm:2 | 2.m*m* |
| 7B | hd06 | 3'3':33 | 3m:3 | 3.m* |
| 7B | hd08 | 4'4':44 | 4mm:4 | 4.m*m* |
| 7B | hd12 | 6'6':66 | 6mm:6 | 6.m*m* |
| 7C | hh08 | 4'4':2'2' | 4mm:2mm | 4*m.m* |
| 7C | hh12 | 6'6':3'3' | 6mm:3m | 6*m.m* |
| 8A | ie08 | 22':20 |  2m: 2m: |  .2*m* .2*m* |
| 8A | ie12 | 23':30 |  m: m: |  .m* .m* |
| 8B | ig08 | 22':222 |  2m:222 2m:222 |  *2.m* *2.m* |
| 8B | ig12 | 23':322 |  m:32 m:32 |  *m* *m* |
| 8C | ih08 | 22':2'2' |  2m:2mm 2m:2mm |  *2*m. *2*m. |
| 8C | Ih12 | 23':3'3' |  m:3m m:3m |  *m. *m. |
| 9A | jf08 | 2'2'2':21' | mmm:2/m | m*m*m. |
| 9A | jf12 | 3'2'2':31' |  m2: m2: |  .m*2* .m*2* |
| 9A | jf16 | 4'2'2':41' | 4/mmm:4/m | 4./m.m*m* |
| 9A | jf24 | 6'2'2':61' | 6/mmm:6/m | 6./m.m*m* |
| 9B | jg08 | 2'2'2':222 | mmm:222 | m*m*m* |
| 9B | jg12 | 3'2'2':322 |  m2:32 m2:32 |  *m*2. *m*2. |
| 9B | jg16 | 4'2'2':422 | 4/mmm:422 | 4./m*m*m* |
| 9B | jg24 | 6'2'2':622 | 6/mmm:622 | 6./m*m*m* |
| 9C | jh08 | 2'2'2':2'2' | mmm:2mm | m.m.m* |
| 9C | jh12 | 3'2'2':3'3' |  m2:3m m2:3m |  *m.2* *m.2* |
| 9C | jh16 | 4'2'2':4'4' | 4/mmm:4mm | 4./m*m.m. |
| 9C | jh24 | 6'2'2':6'6' | 6/mmm:6mm | 6./m*m.m. |
| 9D | ji16 | 4'2'2':22' | 4/mmm: 2m 2m | 4*/m*m*m. |
| 9D | ji24 | 6'2'2':23' | 6/mmm: m m | 6*/m*m*m. |
| 9E | jj16 | 4'2'2':2'2'2' | 4/mmm:mmm | 4*/m.m.m* |
| 9E | jj24 | 6'2'2':3'2'2' | 6/mmm: m2 m2 | 6*/m.m.m* |
| #10 | kg12 | 332:222 | 23:222 | 23# |
| #11 | lj24 | 32':2'2'2' | m :mmm :mmm | m # # |
| 12 | lk24 | 32':332 | m :23 :23 | m* . . |
| 13 | mk24 | 3'3'2':332 |  3m:23 3m:23 |  *3.m* *3.m* |
| 14 | nk24 | 432:332 | 432:23 | 4*3.2* |
| 15A | ol48 | 4'3'2':32' | m m:m m:m | m. .m* .m* |
| 15B | om48 | 4'3'2':3'3'2' | m m: m: 3m 3m | m* .m. .m. |
| 15C | on48 | 4'3'2':432 | m m:432 m:432 | m* .m* .m* |
The identification system used in column 2 of Table A.2 is based upon the
15 topology families (a-o) of regular elliptic orbifolds illustrated in
Fig. 2.3.
The special case "low cyclic" groups of 1, 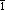 , and
m are somewhat atypical and thus arranged as separate families (a-c)
with the typical cyclic group members in (d-f) and (g-j) from dihedral
groups. In addition, the cubic groups add 6 additional topology
families (k-o) with (k-m) from tetrahedral and (n-o) from octahedral
groups.
, and
m are somewhat atypical and thus arranged as separate families (a-c)
with the typical cyclic group members in (d-f) and (g-j) from dihedral
groups. In addition, the cubic groups add 6 additional topology
families (k-o) with (k-m) from tetrahedral and (n-o) from octahedral
groups.
Matrix Structure in Color Orbifolds Table
Interconnections between all these families require a 13 x 13 matrix which has a partitioning into triangular matrices as shown in Table A.3.
| Groups | Subgroups | ||||
|---|---|---|---|---|---|
| Low Cyclic (a-c) |
Cyclic (d-f) |
Dihedral (g-j) |
Tetrahedral (k-m) |
Octahedral (n-o) |
|
| Low Cyclic (a-c) |
1-2 | ||||
| Cyclic (d-f) |
#3A #(4A) #5A-5B |
3B 4B #5C-5E |
|||
| Dihedral (g-j) |
7A | 6A 7B 9A |
6B 7C-8C 9B-9E |
||
| Tetrahedral (k-m) |
#(10-11) | 12-13 | |||
| Octahedral (n-o) |
14 | 15A-15C | |||
The categories containing tricolor orbifolds are marked with a # if all the members are tricolor.
Omitting the low cyclic column temporarily since first members of a series often behave abnormally, we basically have two submatrices with elements (d-j) and (k-o) connected by the tricolor orbifolds (10-11). This means that the only way to get to the cubic orbifolds from the lower members is through a 3-sheeted cover corresponding to the diagonal 3-axis in the cubic space groups.
The elements within the (d-j) submatrix further decomposes into identical submatrices for the octahedral-tetragonal and trigonal-hexagonal crystallographic families, connected only through 3-sheeted covers. This relationship is also readily apparent in Fig. A.2. The color orbifold drawings in Appendix B are arranged according to these observations.
Bicolor Space Group Wyckoff Sites
As an example application of bicolor point groups and space groups to
the confirmation of Euclidean 3-orbifolds, we examine the Wyckoff sites
for the seven index-two subgroups for Im m (#229)
using Table 12 of the Appendix in Koptsik's book, which lists all
the Wyckoff sites for the 1191 bicolor space groups. Using the notation of
Opechowski and Guccione, the bicolor groups in the last four
columns of Table A.4 have an antisymmetric lattice, Ip, in which the
generator for the body centering vector has negative parity. The last
four subgroups have the same point group, m
m (#229)
using Table 12 of the Appendix in Koptsik's book, which lists all
the Wyckoff sites for the 1191 bicolor space groups. Using the notation of
Opechowski and Guccione, the bicolor groups in the last four
columns of Table A.4 have an antisymmetric lattice, Ip, in which the
generator for the body centering vector has negative parity. The last
four subgroups have the same point group, m m,
as Im
m,
as Im m while the
first three have the same lattice, I, as Im
m while the
first three have the same lattice, I, as Im m
but different point groups.
m
but different point groups.
The symbols listed in the second line of the column headers for Table A.4 of this section are for the 2-elliptic suborbifolds from the second half (suborbifold) of the orbifold-suborbifold notation in column 2 of Table A.2. The orbifold part of the orbifold-suborbifold notation is in the row labels of Table A.4. The row sequence in Table A.4 was arranged to match the sequence of peak/pass/pale/pit critical points in the body-centered cubic critical net shown in Fig. A.1 (see Introduction to Critical Nets).
 m
m
| Orbifold | Suborbifold | |||||||
|---|---|---|---|---|---|---|---|---|
Wyckoff Site |
Im  m m229 |
Im m mI432 211 |
Im m mIm  204 |
Im m mI  3m 3m217 |
Ipm m mPn  n n222 |
Ipm m mPn  m m224 |
Ipm m mPm  n n223 |
Ipm m mPm  m m221 |
| 48 k | 1' | 1 | 1 | 1' | 1 | 1' | 1 | 1' |
| 12 e | 4'4' | 44 | 2'2' | 2'2' | 44 | 2'2' | 2'2' | 4'4' |
| 2 a | 4'3'2' | 432 | 32' | 3'3'2' | 432 | 3'3'2' | 32' | 4'3'2' |
| 16 f | 3'3' | 33 | 33 | 3'3' | 33 | 3'3' | 33 | 3'3' |
| 8 c | 23' | 322 | 30 | 3'3' | 30 | 23' | 322 | 3'3' |
| 48 i | 22 | 22 | 1 | 1 | 1 | 22 | 22 | 1 |
| 12 d | 22' | 222 | 2'2' | 20 | 20 | 222 | 22' | 2'2' |
| 24 g | 2'2' | 22 | 2'2' | 22 | 22 | 22 | 2'2' | 2'2' |
| 6 b | 4'2'2' | 422 | 2'2'2' | 22' | 422 | 22' | 2'2'2' | 4'2'2' |
| 24 h | 2'2' | 22 | 1' | 1' | 22 | 1' | 1' | 2'2' |
| 48 j | 1' | 1 | 1' | 1 | 1 | 1 | 1' | 1' |
Note that whenever an element of Table A.4 is the same within the orbifold and suborbifold, it is split into two parts in the suborbifold drawing (shown in Fig. A.1). The Table A.4 entries provide an independent confirmation of the space group orbifold critical nets shown in Fig. A.1, which we had derived from the eight individual space groups by distorting the asymmetric unit (fundamental domain) of each space group to bring matching points along its boundary into superposition, as illustrated in Fig. 4.1, to produce the orbifold. Note that the orbifolds can contain mirror planes as boundaries and projective planes (RP2 and RP3) as gluing surfaces.

 m.
m.Fig. A.1 suggests that a space group orbifold can also be derived as a suborbifold of a parent orbifold when the corresponding two groups have a group/normal-subgroup relationship. The table of Wyckoff sites given in Koptsik's Shubnikov groups book for a bicolor space group is much more convenient for data base archiving than having to match up the inconsistently labeled sets of Wyckoff sites from two different space groups from the International Tables, particularly if the goal is computer automation of the process based on table lookup. However a problem with the Koptsik tabulation is frequent misprints or errors. To be really useful, the Shubnikov groups should be rederived computationally and used in a database environment.
Orbifold/Sub-orbifold Graph
Fig. A.2, which was derived from Fig 10.3.2 in the International Tables for Crystallography (Hahn, 1995), shows the orbifold/sub-orbifold graph for elliptic-2 orbifolds. The graph nodes are labeled with the notation of Fig. 2.3 except that prefix letters have been dropped and nine of the nodes have new notation. The low cyclic s become: S -> 1, RP -> 0, D -> 1', and the cyclic changes are D2 -> 21', D3 -> 31', D4 -> 41', D6 -> 61'. We will use this notation for the color groups also.
Visualize the graph as having three-dimensional character with three subgraphs parallel to the x-y plane layered over each other along the z axis. The bottom layer contains the low-cyclic a,b,c orbifolds (i.e. 1, 0 and 1') in the left front, the cyclic orthorhombic-tetragonal (COT) d,e,f orbifolds behind them away from the viewer, and the dihedral orthorhombic-tetragonal (DOT) orbifolds to the right. The middle layer contains the trigonal-hexagonal orbifolds with the CTH to the left and the DTH to the right. The top layer contains the cubic orbifolds from the tetrahedral (front three) and octahedral (back two) point groups.
Note that COT+DOT and CTH+DTH have identical connectivity within their planes, which is an important relation making 3- and 6-fold axes interchangeable with corresponding 2- and 4-fold axes throughout orbifolds in these layers. This is the main reason for giving the low cyclic groups a special classification.
All solid lines leaving the bottom of an orbifold connect to the top of in index-2 normal suborbifold. The heavy square-dotted lines connect to index-3 normal suborbifolds and the light square-dotted lines connect to index-3 non-normal suborbifolds. The light dashed lines between the top and middle layers connect to index-4 non-normal suborbifolds. Note that all index-2 connections are in a layer and point to the left and/or forward. Other connections tend to point down, left, and/or forward but there are a few exceptions. These observations may relate to subgroup lattices for the crystallographic groups.
Page last revised: July 23, 1996

Figure A.2. Group/Subgroup Graph for Crystallographic Point Groups.
(solid lines = index 2 normal; heavy dotted lines = index 3 normal;
light dotted lines = index 3 conjugate; light dashed = index 4 conjugate)
 Appendix B. Elliptic 2-Orbifolds Covers
Appendix B. Elliptic 2-Orbifolds Covers
 6. Cubic Space Group/Subgroup Families
6. Cubic Space Group/Subgroup Families
 Crystallographic Topology Home Page
Crystallographic Topology Home Page